Repetition and nesting are widespread themes in many cellular automata. But as we saw in the previous chapter, it is also possible for cellular automata to produce patterns that seem in many respects random. And out of the 256 rules discussed here, it turns out that 10 yield such apparent randomness. There are three basic forms, as illustrated on the facing page.

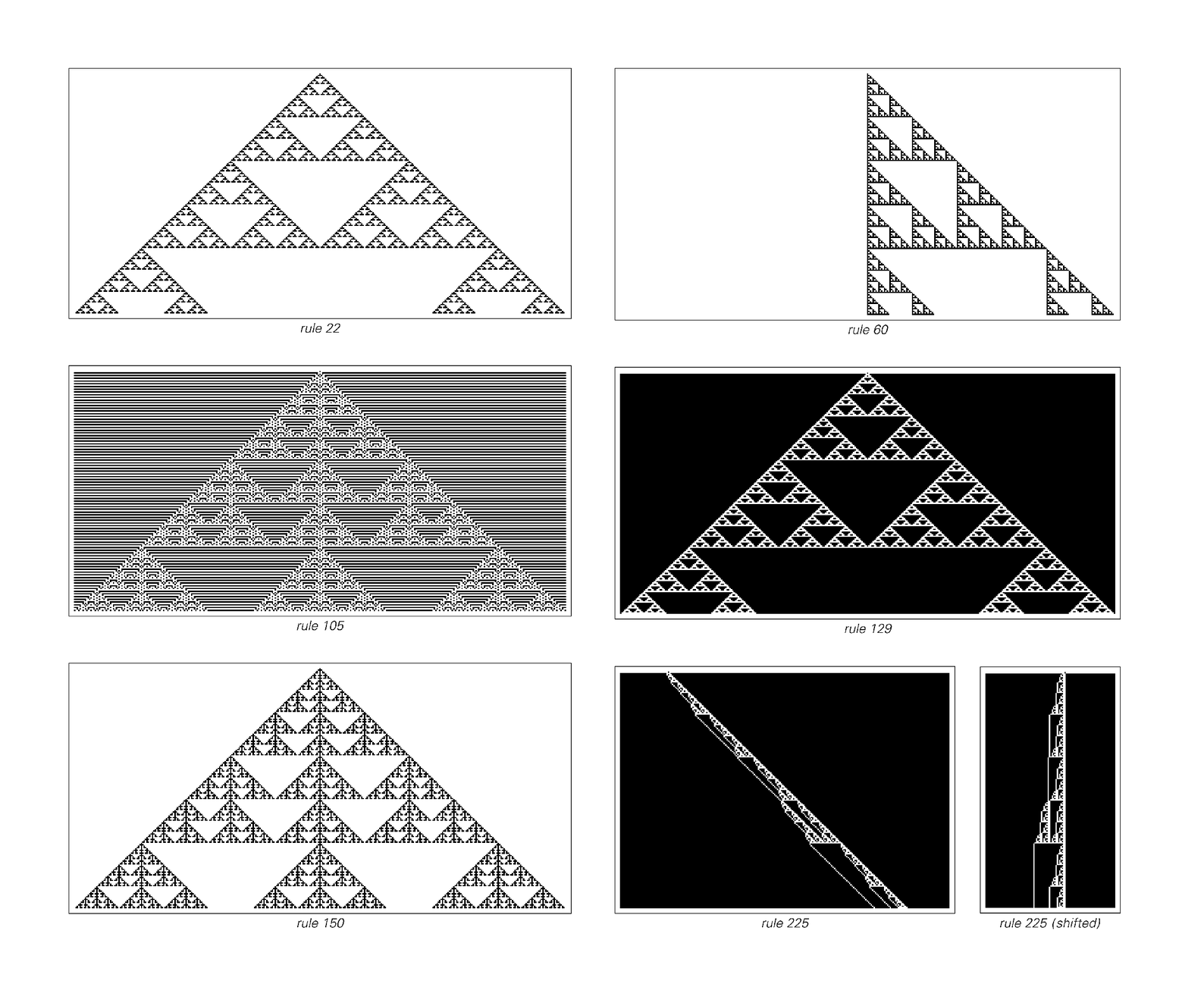
Examples of cellular automata that produce nested or fractal patterns. Rule 22—like rule 90 from page 26—gives a pattern with fractal dimension Log[2,3] ≃ 1.58; rule 150 gives one with fractal dimension Log[2, 1+Sqrt[5]] ≃ 1.69. The width of the pattern obtained from rule 225 increases like the square root of the number of steps.