So what about three-dimensional cellular automata? It is straightforward to generalize the setup for two-dimensional rules to the three-dimensional case. But particularly on a printed page it is fairly difficult to display the evolution of a three-dimensional cellular automaton in a way that can readily be assimilated.

Pages 182 and 183 do however show a few examples of three-dimensional cellular automata. And just as in the two-dimensional case, there are some specific new phenomena that can be seen. But overall it seems that the basic kinds of behavior produced are just the same as in one and two dimensions. And in particular, the basic phenomenon of complexity does not seem to depend in any crucial way on the dimensionality of the system one looks at.

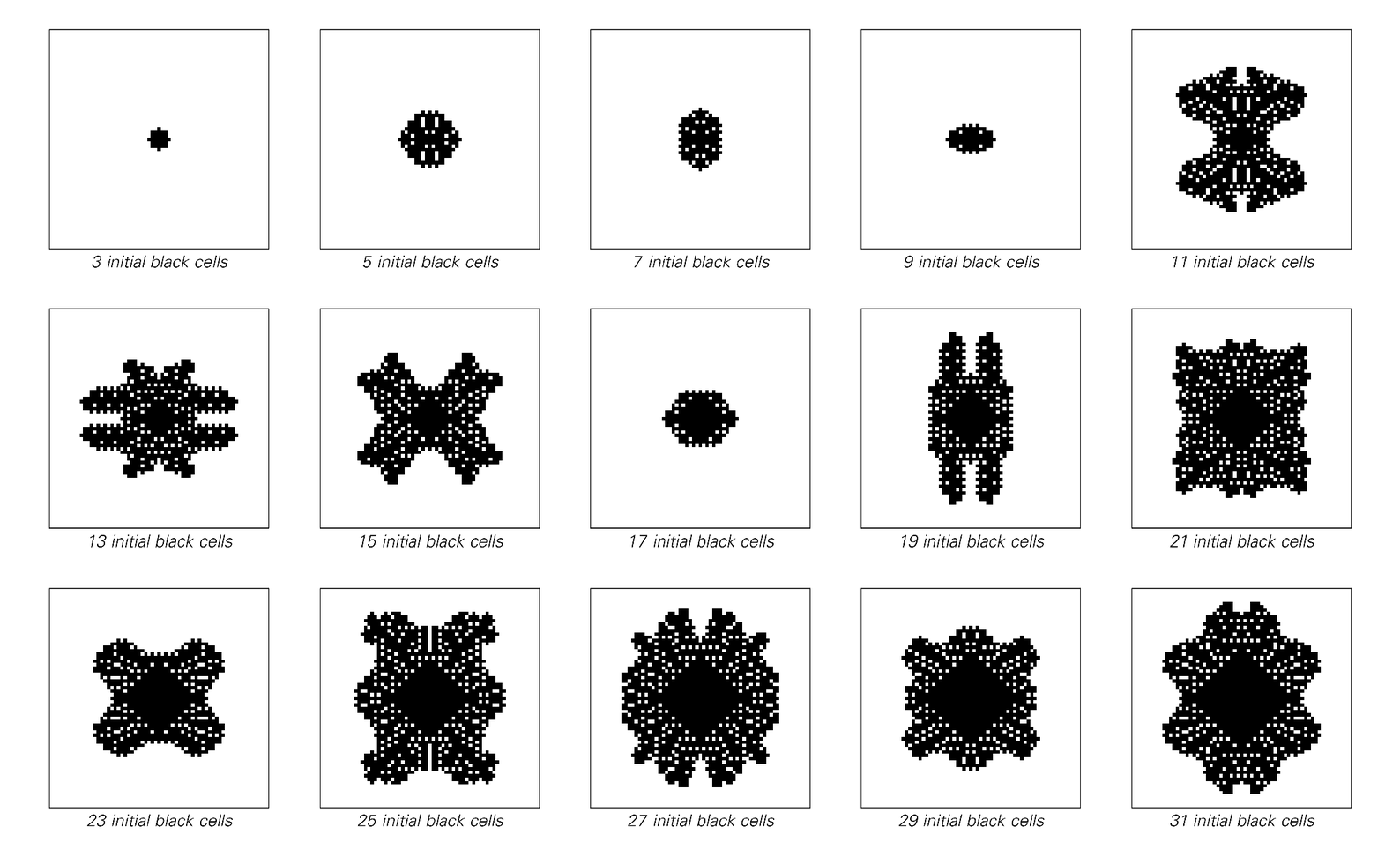
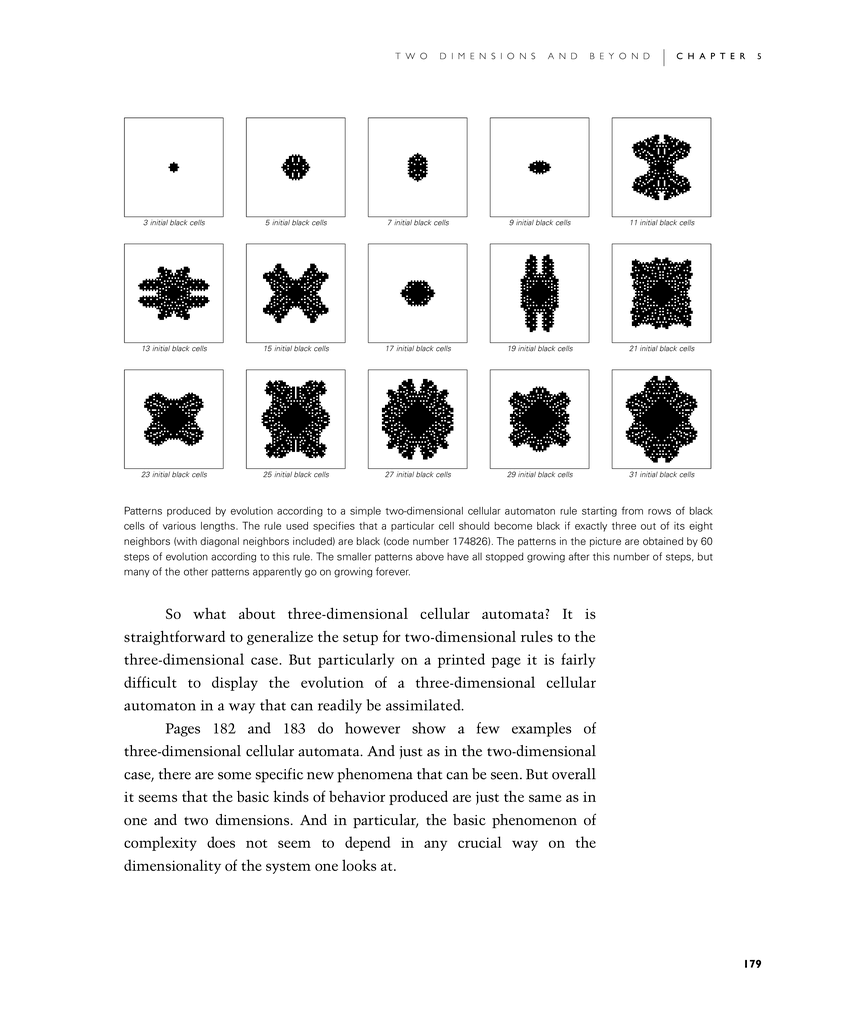
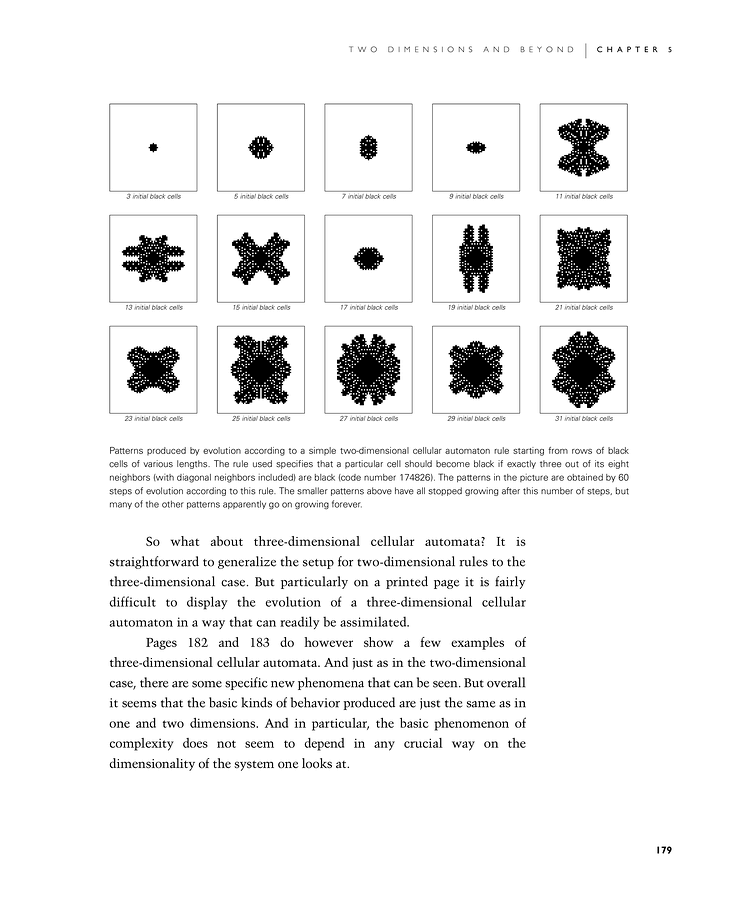
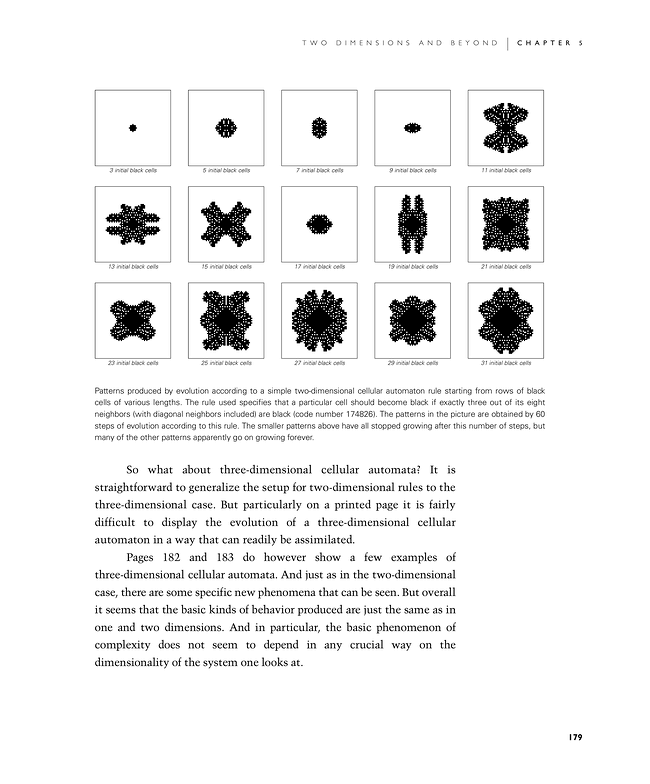
Patterns produced by evolution according to a simple two-dimensional cellular automaton rule starting from rows of black cells of various lengths. The rule used specifies that a particular cell should become black if exactly three out of its eight neighbors (with diagonal neighbors included) are black (code number 174826). The patterns in the picture are obtained by 60 steps of evolution according to this rule. The smaller patterns above have all stopped growing after this number of steps, but many of the other patterns apparently go on growing forever.