The Notion of Reversibility
At any particular step in the evolution of a system like a cellular automaton the underlying rule for the system tells one how to proceed to the next step. But what if one wants to go backwards? Can one deduce from the arrangement of black and white cells at a particular step what the arrangement of cells must have been on previous steps?
All current evidence suggests that the underlying laws of physics have this kind of reversibility. So this means that given a sufficiently precise knowledge of the state of a physical system at the present time, it is therefore possible to deduce not only what the system will do in the future, but also what it did in the past.
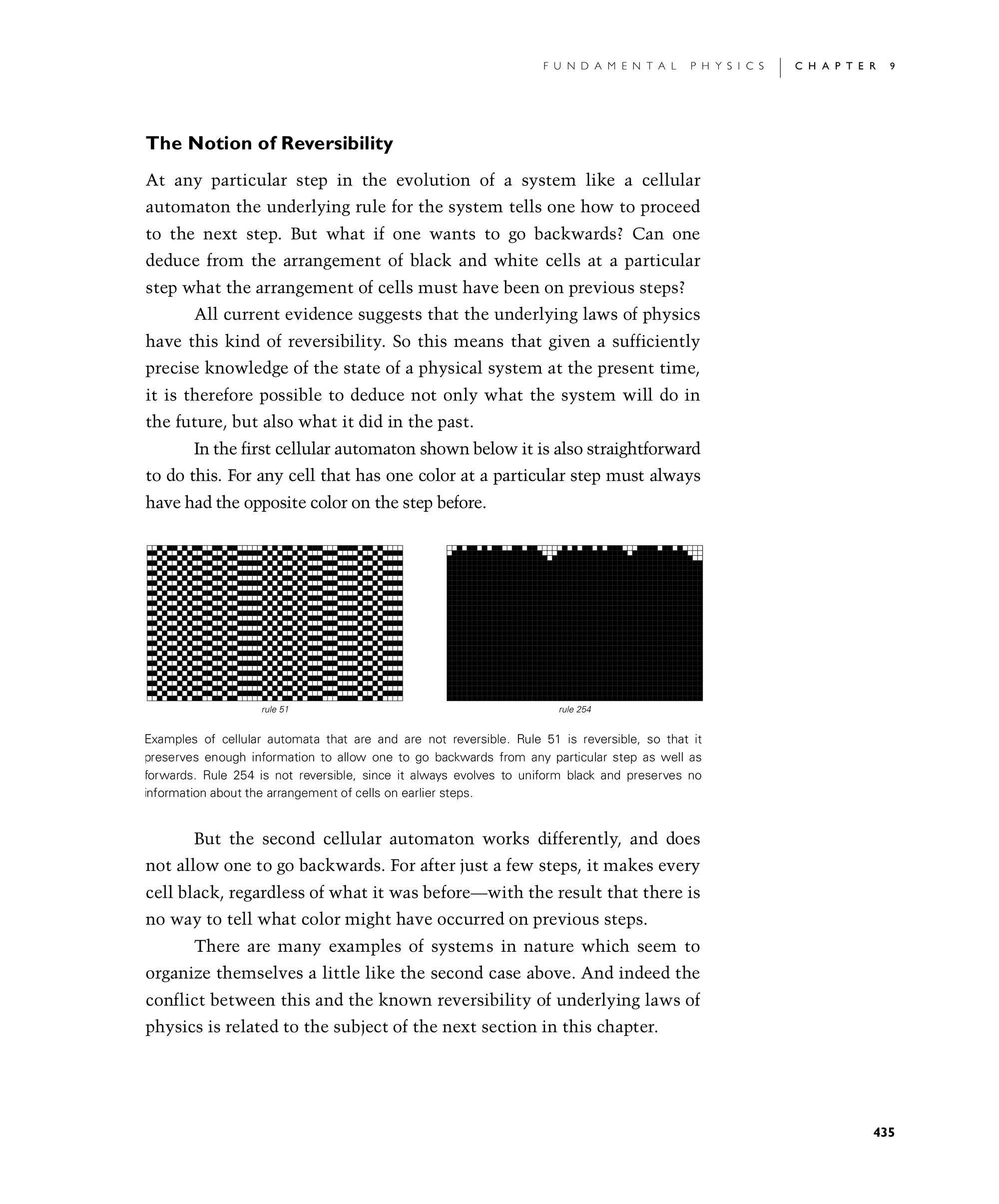
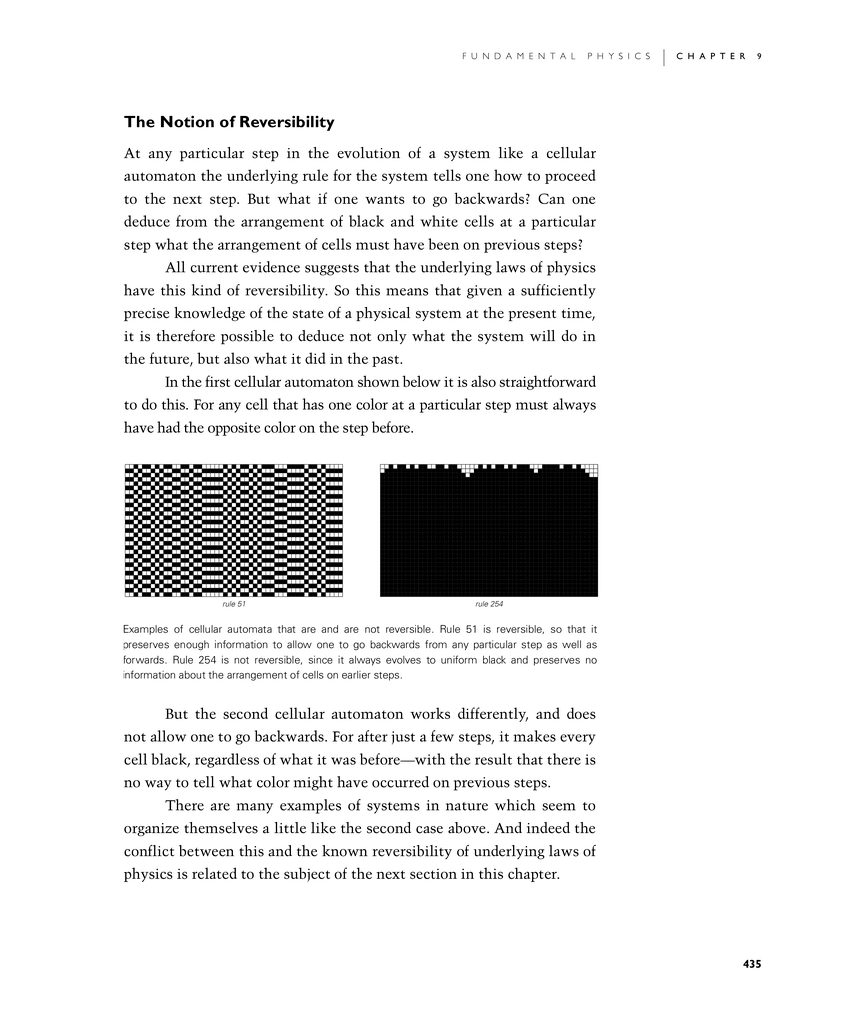
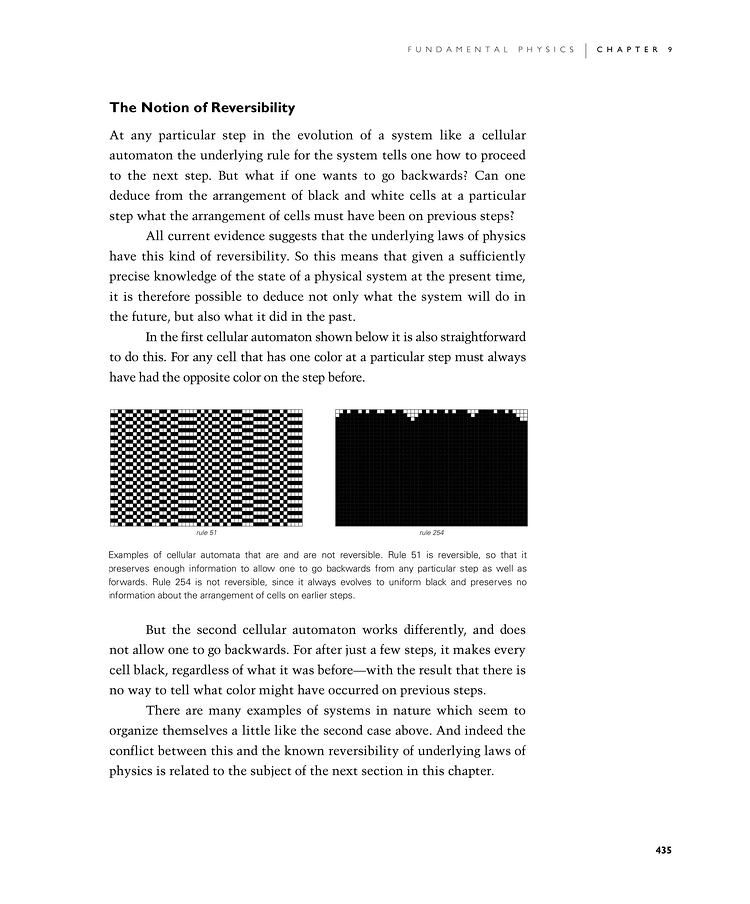
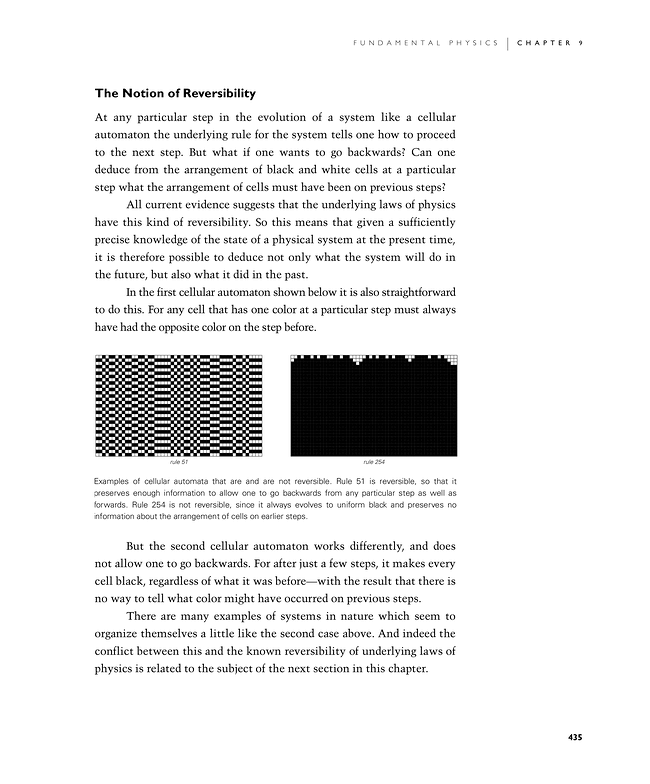
In the first cellular automaton shown below it is also straightforward to do this. For any cell that has one color at a particular step must always have had the opposite color on the step before.
But the second cellular automaton works differently, and does not allow one to go backwards. For after just a few steps, it makes every cell black, regardless of what it was before—with the result that there is no way to tell what color might have occurred on previous steps.
There are many examples of systems in nature which seem to organize themselves a little like the second case below. And indeed the conflict between this and the known reversibility of underlying laws of physics is related to the subject of the next section in this chapter.
Examples of cellular automata that are and are not reversible. Rule 51 is reversible, so that it preserves enough information to allow one to go backwards from any particular step as well as forwards. Rule 254 is not reversible, since it always evolves to uniform black and preserves no information about the arrangement of cells on earlier steps.