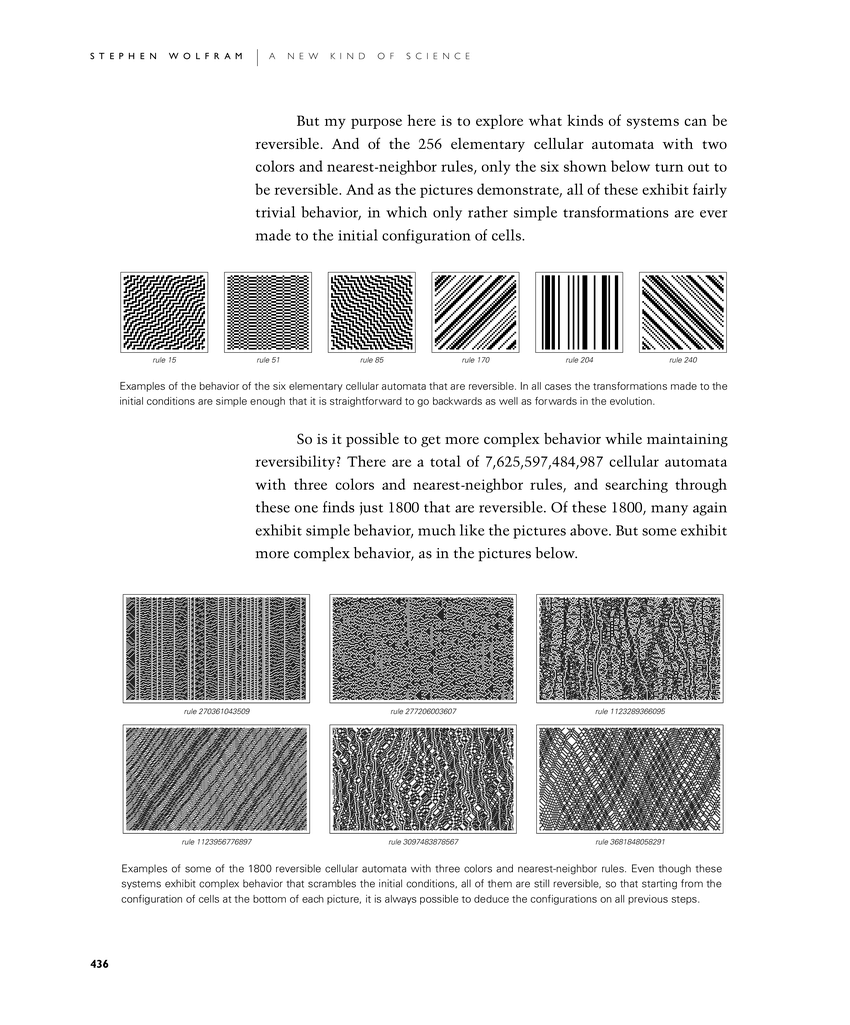
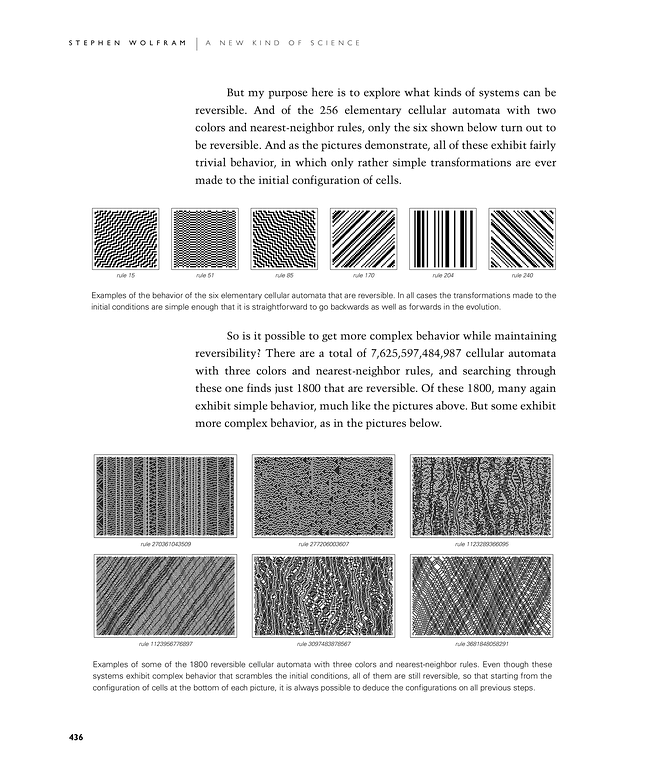
But my purpose here is to explore what kinds of systems can be reversible. And of the 256 elementary cellular automata with two colors and nearest-neighbor rules, only the six shown below turn out to be reversible. And as the pictures demonstrate, all of these exhibit fairly trivial behavior, in which only rather simple transformations are ever made to the initial configuration of cells.
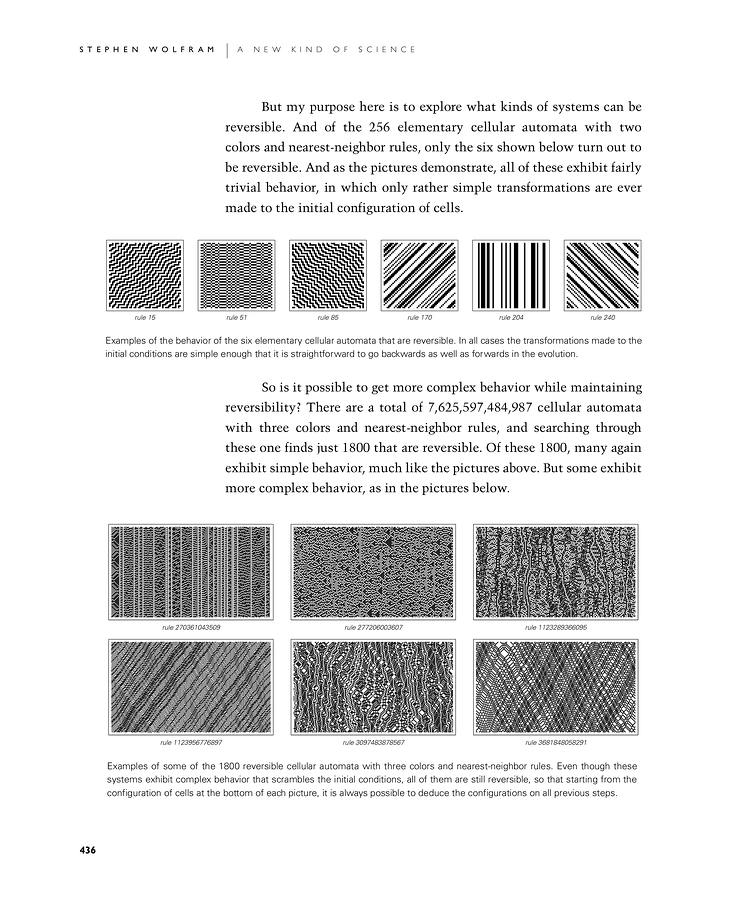
So is it possible to get more complex behavior while maintaining reversibility? There are a total of 7,625,597,484,987 cellular automata with three colors and nearest-neighbor rules, and searching through these one finds just 1800 that are reversible. Of these 1800, many again exhibit simple behavior, much like the first set of pictures below. But some exhibit more complex behavior, as in the second set of pictures below.
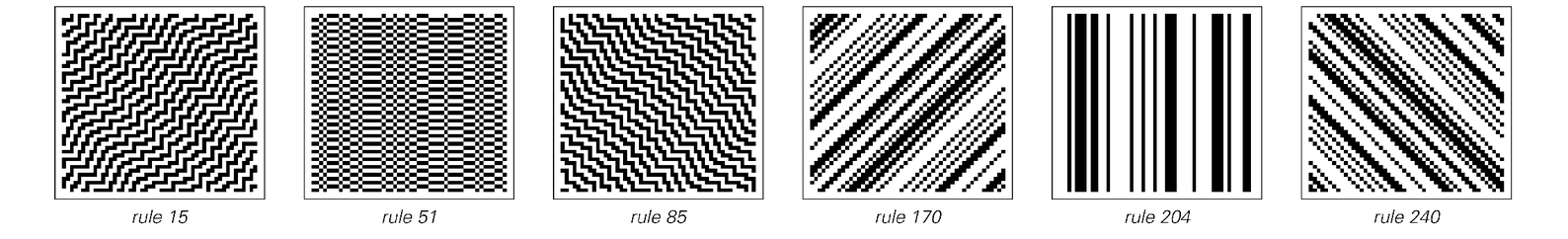
Examples of the behavior of the six elementary cellular automata that are reversible. In all cases the transformations made to the initial conditions are simple enough that it is straightforward to go backwards as well as forwards in the evolution.
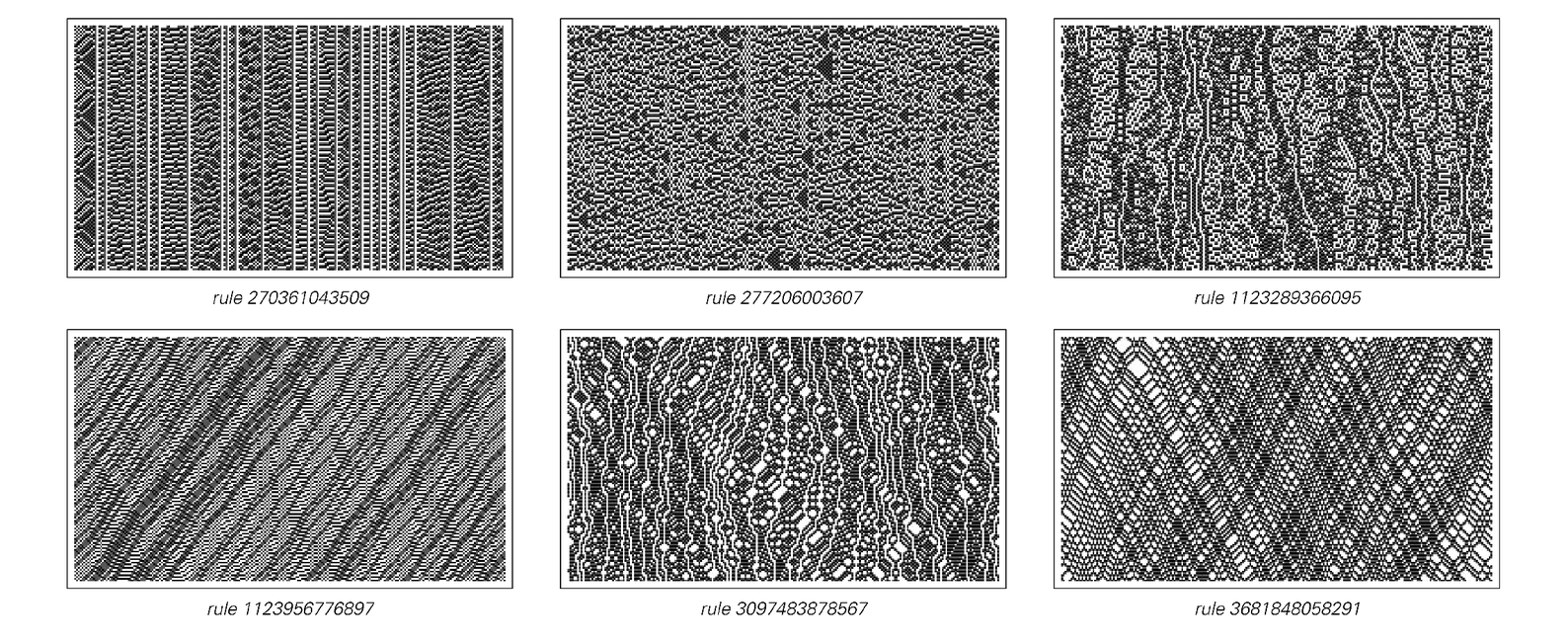
Examples of some of the 1800 reversible cellular automata with three colors and nearest-neighbor rules. Even though these systems exhibit complex behavior that scrambles the initial conditions, all of them are still reversible, so that starting from the configuration of cells at the bottom of each picture, it is always possible to deduce the configurations on all previous steps.