The second set of pictures below show examples of other cellular automata that exhibit the same basic phenomenon. In each case the initial conditions are random, but the system nevertheless quickly organizes itself to become either uniformly white or uniformly black.
The facing page shows cellular automata that exhibit slightly more complicated behavior. Starting from random initial conditions, these cellular automata again quickly settle down to stable states. But now these stable states are not just uniform in color, but instead involve a collection of definite structures that either remain fixed on successive steps, or repeat periodically.
So if they have simple underlying rules, do all cellular automata started from random initial conditions eventually settle down to give stable states that somehow look simple?
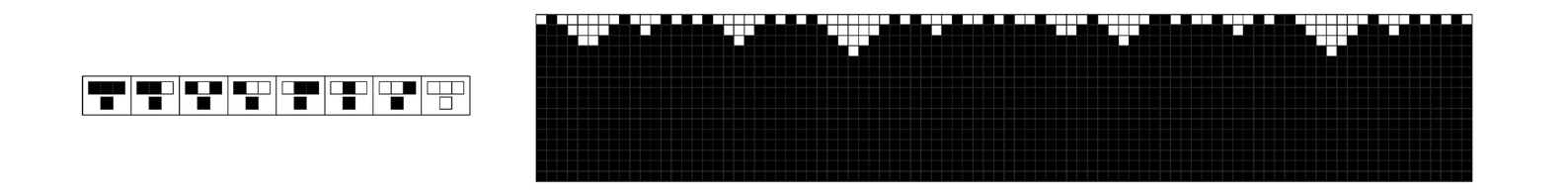
A cellular automaton that evolves to a simple uniform state when started from any random initial condition. The rule in this case was first shown on page 24, and is number 254 in the scheme described on page 53. It specifies that a cell should become black whenever either of its neighbors is already black.
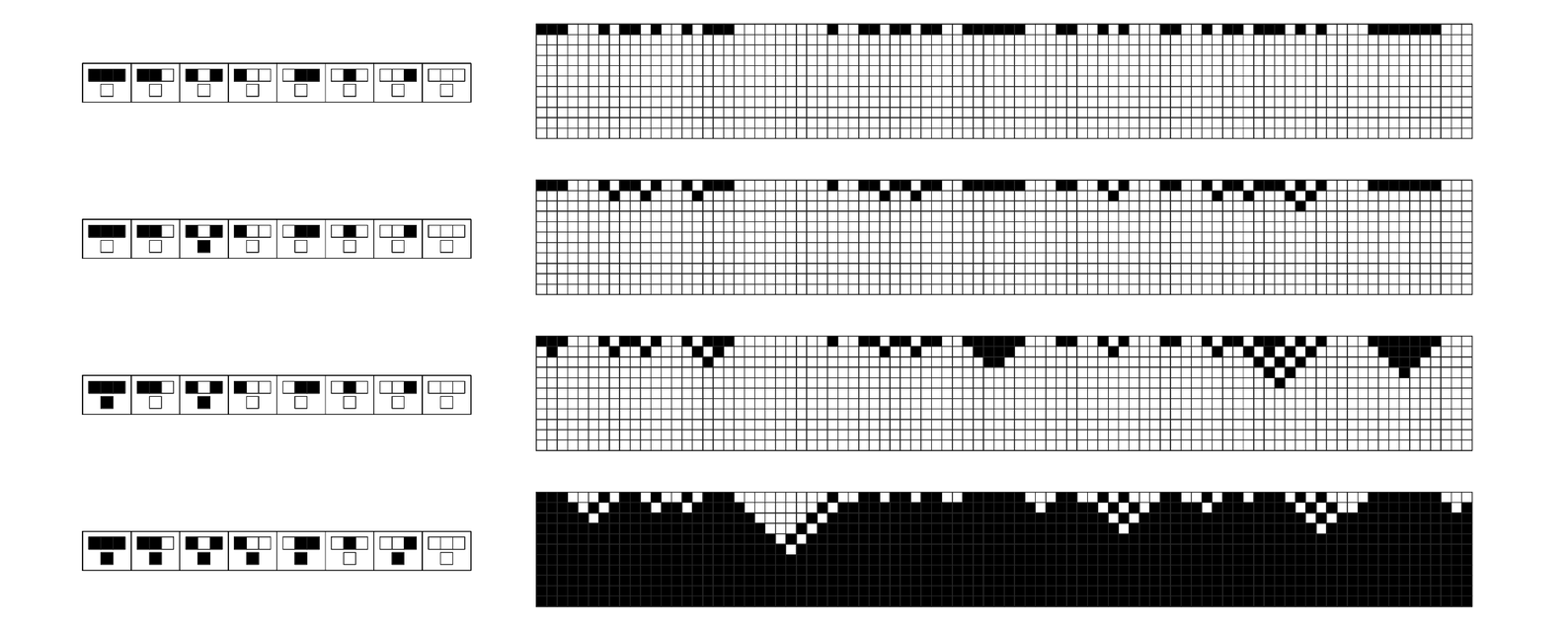
Four more examples of cellular automata that evolve from random initial conditions to completely uniform states. The rules shown here correspond to numbers 0, 32, 160 and 250.