But there is still an important constraint on the behavior: even though black and gray cells may in effect move around randomly, their total number must always be conserved. And this means that if one looks at the total average density of colored cells throughout the system, it must always remain the same. But local densities in different parts of the system need not—and in general they will change as colored cells flow in and out.

The pictures below show what happens with four different rules, starting with higher density in the middle and lower density on the sides. With rules (a) and (b), each different region effectively remains separated forever. But with rules (c) and (d) the regions gradually mix.

As in many kinds of systems, the details of the initial arrangement of cells will normally have an effect on the details of the behavior that occurs. But what the pictures below suggest is that if one looks only at the overall distribution of density, then these details will become largely irrelevant—so that a given initial distribution of density will always tend to evolve in the same overall way, regardless of what particular arrangement of cells happened to make up that distribution.

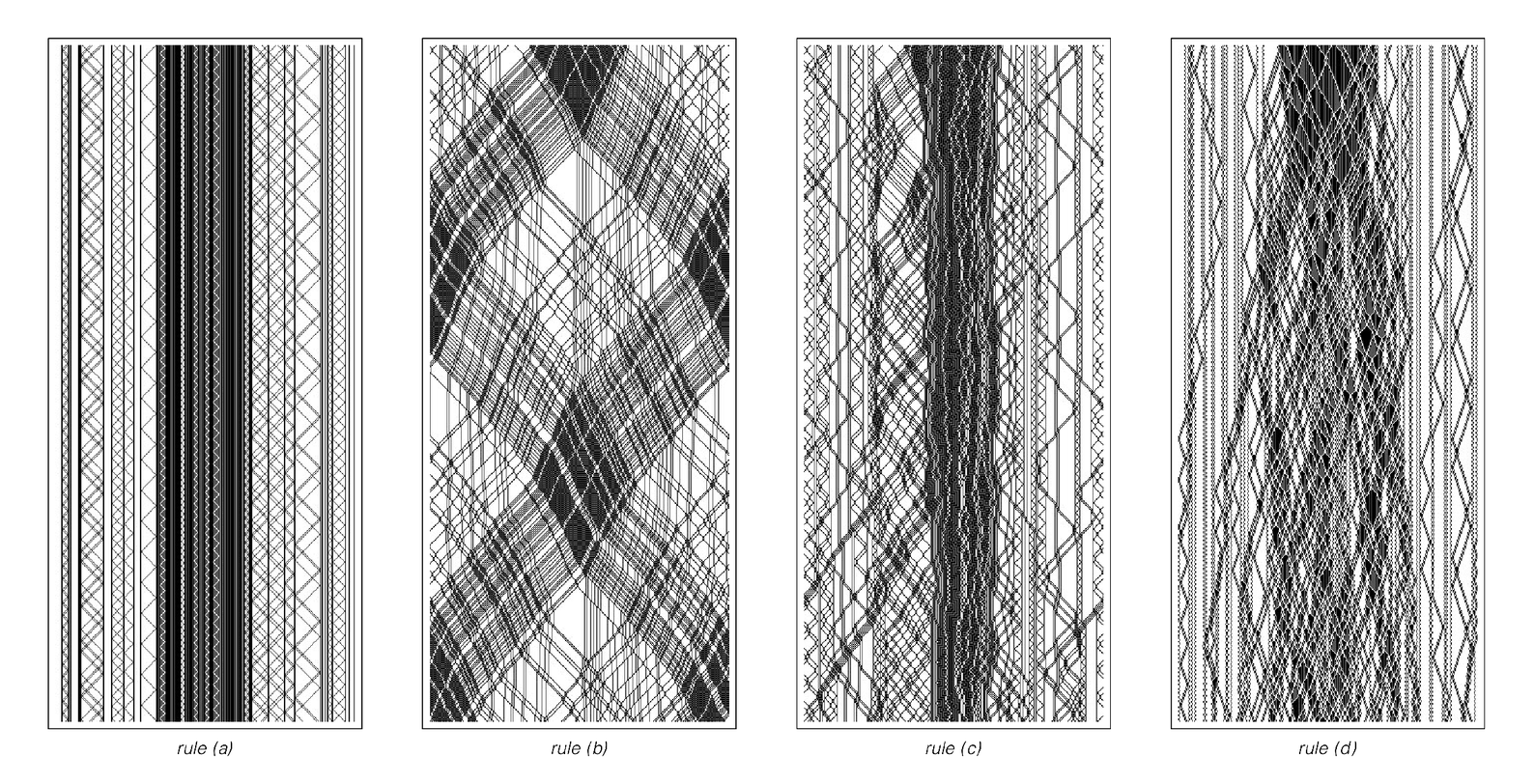
The block cellular automata from previous pages [461, 462] started from initial conditions containing regions of different density. In rules (a) and (b) the regions remain separated forever, but in rules (c) and (d) they gradually diffuse into each other.