samples just the first cell in every 14×7 block of cells, making each domain of repetitive behavior stand out as having a uniform color.
In the detailed behavior of the various localized structures that separate these domains of repetitive behavior there is all sorts of complexity. But what the picture suggests is that at some rough overall level these structures progressively tend to annihilate each other, and in doing so form an approximate nested pattern.
It turns out that this basic process is not restricted to systems which produce simple uniform or repetitive domains. And the pictures below show for example cases where the behavior inside each domain is quite random.
Instead of following simple straight lines, the boundaries of these domains now execute seemingly random walks. But the fact that they annihilate whenever they meet once again tends to lead to an overall nested pattern of behavior.
So what about systems based on constraints? Can these also lead to nesting? In Chapter 5 I showed that they can. But what I found is that whereas at least in principle both uniformity and repetition can be forced fairly easily by constraints, nesting usually cannot be.
At the outset, one might have thought that there would be just one definite mechanism for each type of simple behavior. But what we
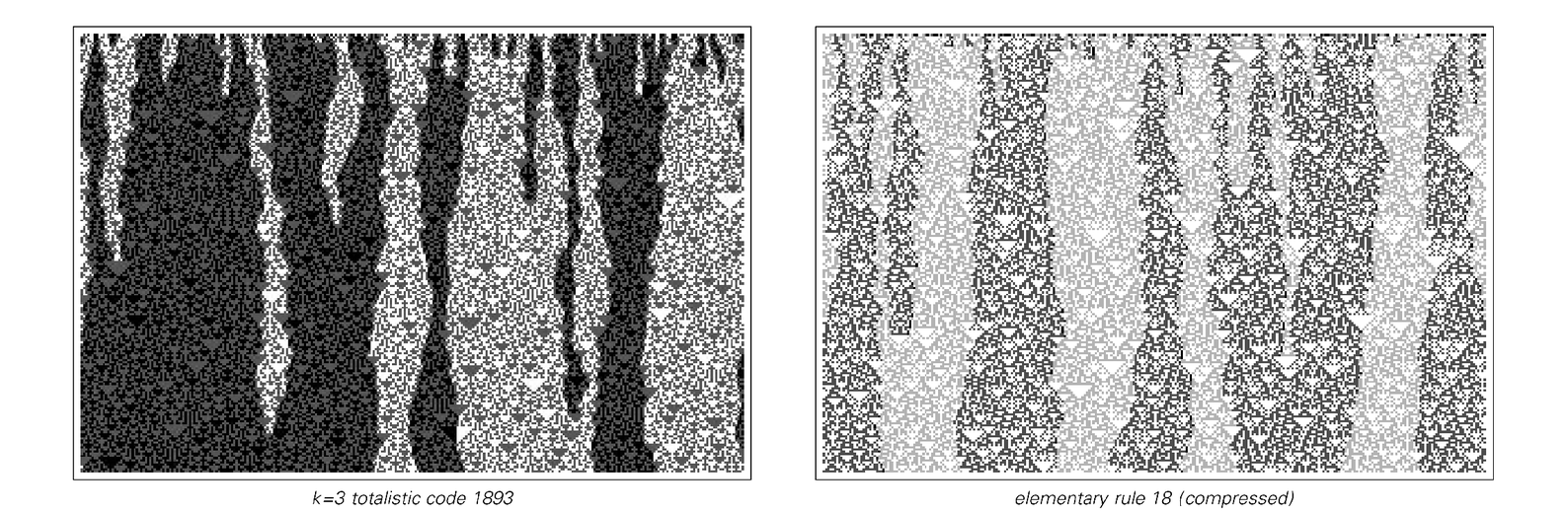
Examples involving domains containing apparent randomness. In the second picture, each element shown represents a 2×2 block of original cells. In both cases, the boundaries between domains appear to follow random walks, annihilating when they meet and thus forming a nested overall pattern.