The shapes produced in each case are very simple, and ultimately consist just of flat facets arranged in a way that reflects directly the structure of the underlying lattice of cells. And many crystals in nature—including for example most gemstones—have similarly simple faceted forms. But some do not. And as one well-known example, snowflakes can have highly intricate forms, as illustrated below.
To a good approximation, all the molecules in a snowflake ultimately lie on a simple hexagonal grid. But in the actual process of snowflake growth, not every possible part of this grid ends up being filled with ice. The main effect responsible for this is that whenever a piece of ice is added to the snowflake, there is some heat released, which then tends to inhibit the addition of further pieces of ice nearby.
One can capture this basic effect by having a cellular automaton with rules in which cells become black if they have exactly one black neighbor, but stay white whenever they have more than one black neighbor. The pictures on the facing page show a sequence of steps in the evolution of such a cellular automaton. And despite the simplicity of its underlying rules, what one sees is that the patterns it produces are strikingly similar to those seen in real snowflakes.
From looking at the behavior of the cellular automaton, one can immediately make various predictions about snowflakes. For example,
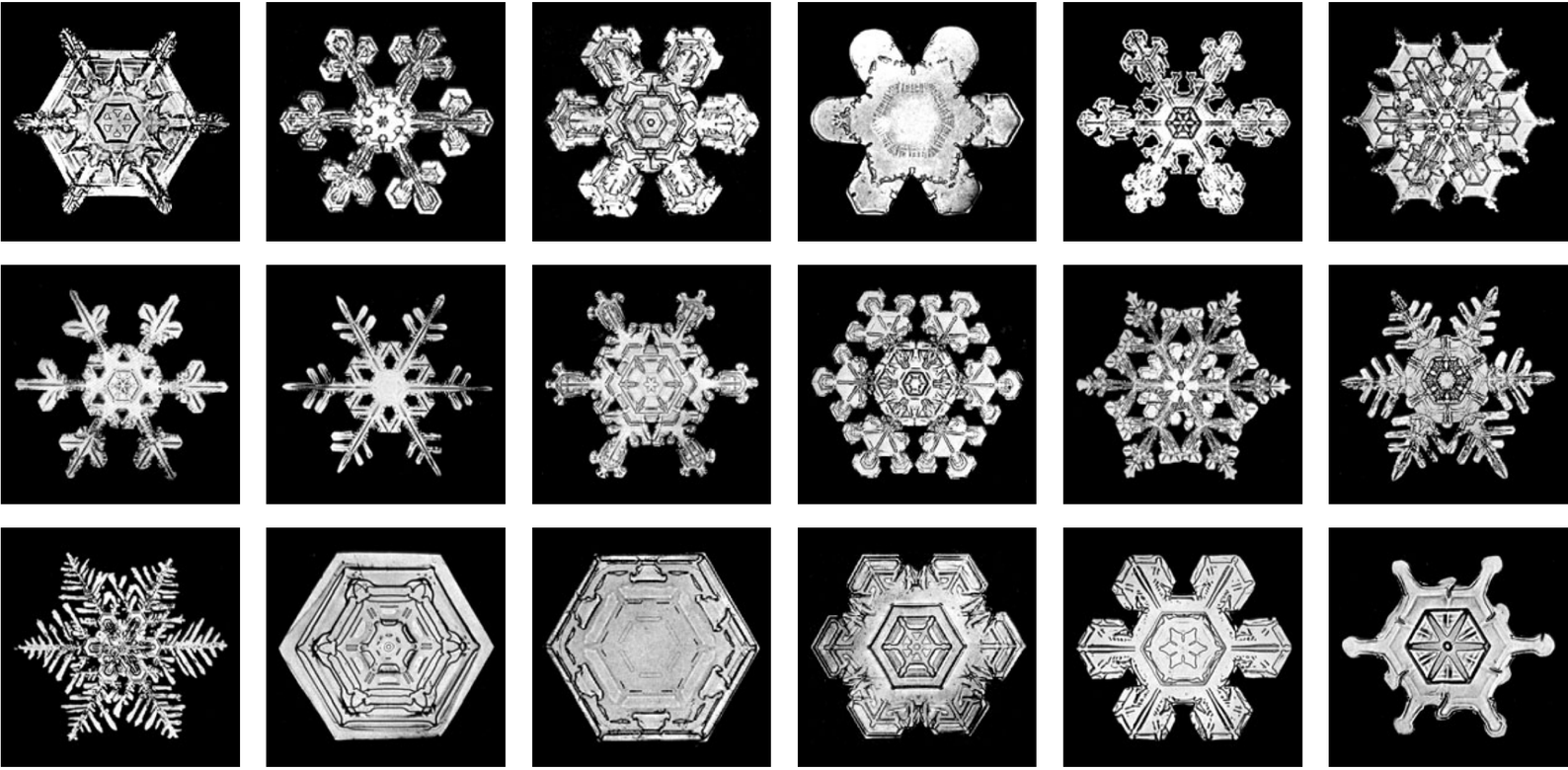
Examples of typical forms of snowflakes. Note that the scales for different pictures are different.