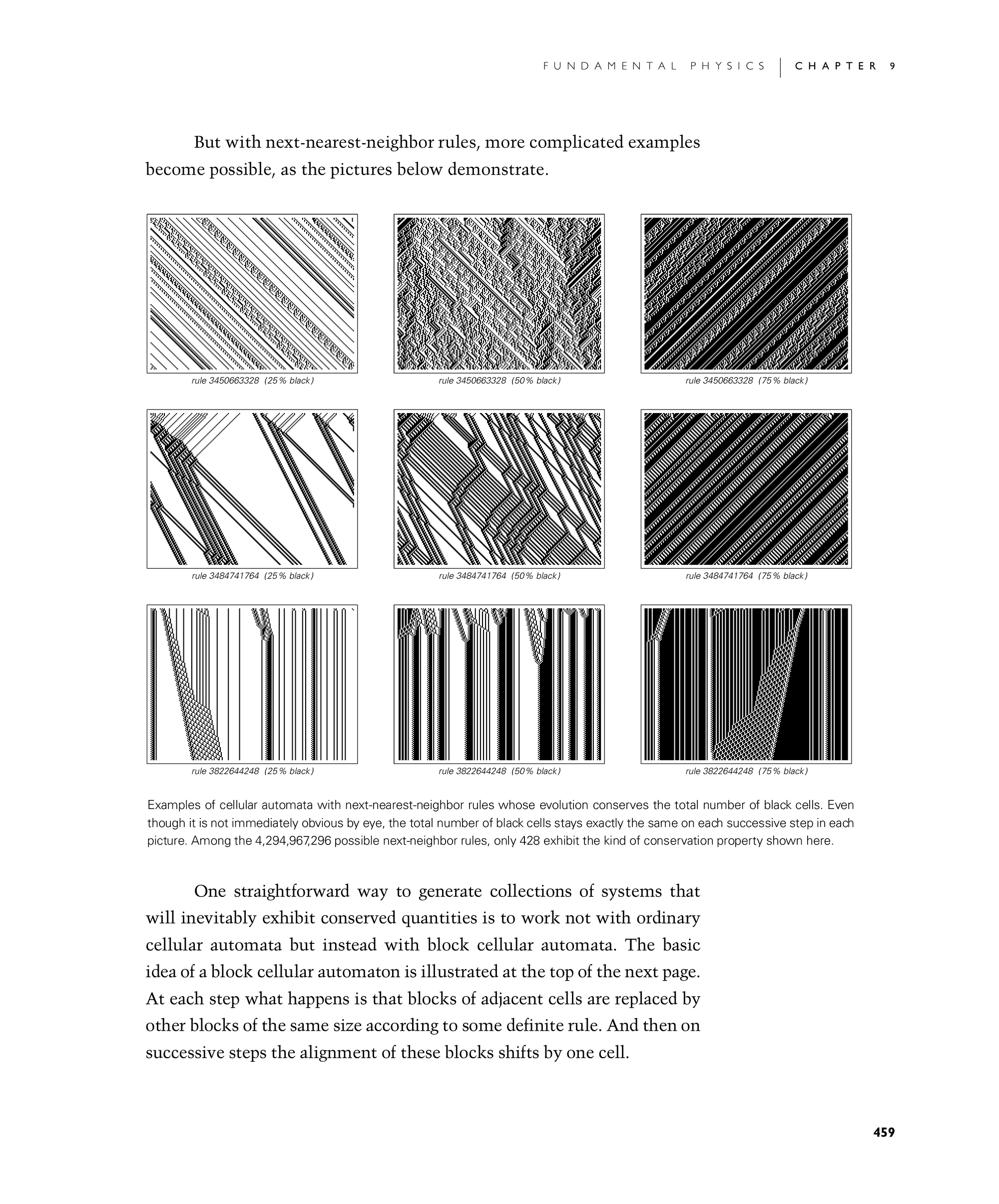
But with next-nearest-neighbor rules, more complicated examples become possible, as the pictures below demonstrate.
One straightforward way to generate collections of systems that will inevitably exhibit conserved quantities is to work not with ordinary cellular automata but instead with block cellular automata. The basic idea of a block cellular automaton is illustrated at the top of the next page. At each step what happens is that blocks of adjacent cells are replaced by other blocks of the same size according to some definite rule. And then on successive steps the alignment of these blocks shifts by one cell.
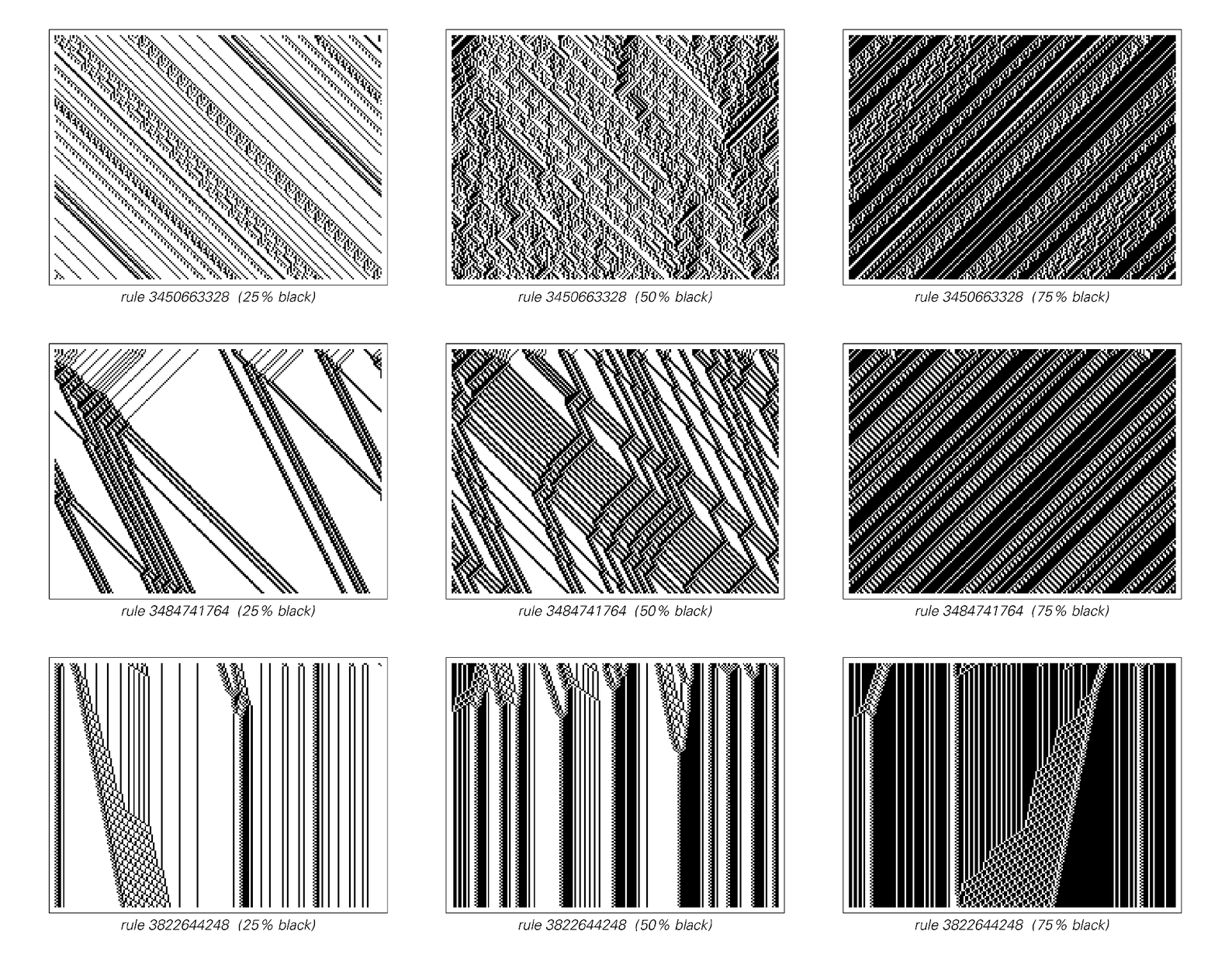
Examples of cellular automata with next-nearest-neighbor rules whose evolution conserves the total number of black cells. Even though it is not immediately obvious by eye, the total number of black cells stays exactly the same on each successive step in each picture. Among the 4,294,967,296 possible next-neighbor rules, only 428 exhibit the kind of conservation property shown here.