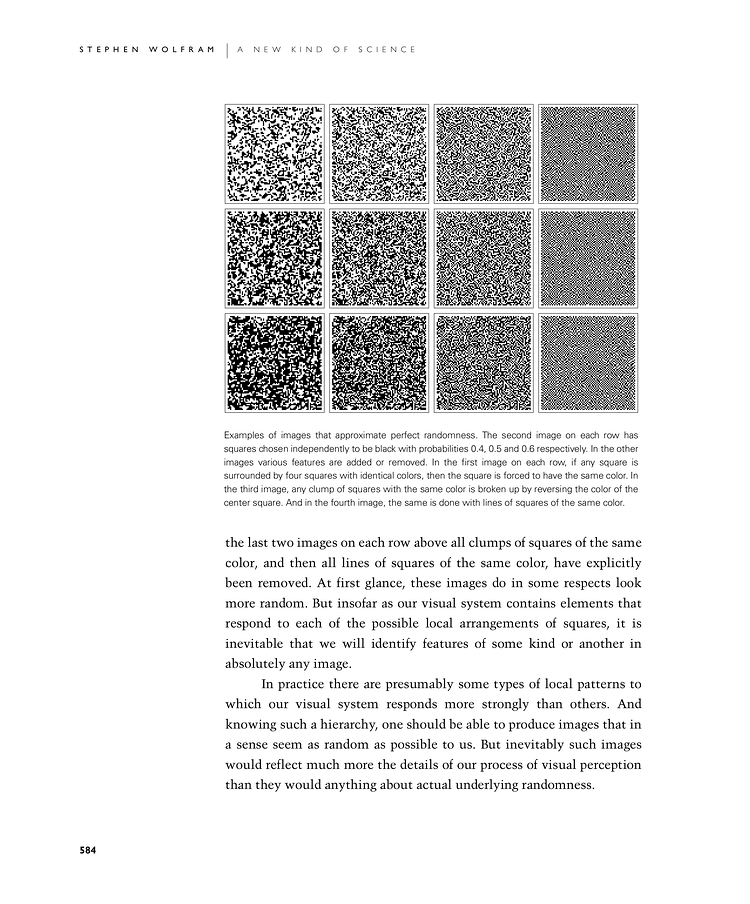
the last two images on each row below all clumps of squares of the same color, and then all lines of squares of the same color, have explicitly been removed. At first glance, these images do in some respects look more random. But insofar as our visual system contains elements that respond to each of the possible local arrangements of squares, it is inevitable that we will identify features of some kind or another in absolutely any image.

In practice there are presumably some types of local patterns to which our visual system responds more strongly than others. And knowing such a hierarchy, one should be able to produce images that in a sense seem as random as possible to us. But inevitably such images would reflect much more the details of our process of visual perception than they would anything about actual underlying randomness.

Examples of images that approximate perfect randomness. The second image on each row has squares chosen independently to be black with probabilities 0.4, 0.5 and 0.6 respectively. In the other images various features are added or removed. In the first image on each row, if any square is surrounded by four squares with identical colors, then the square is forced to have the same color. In the third image, any clump of squares with the same color is broken up by reversing the color of the center square. And in the fourth image, the same is done with lines of squares of the same color.