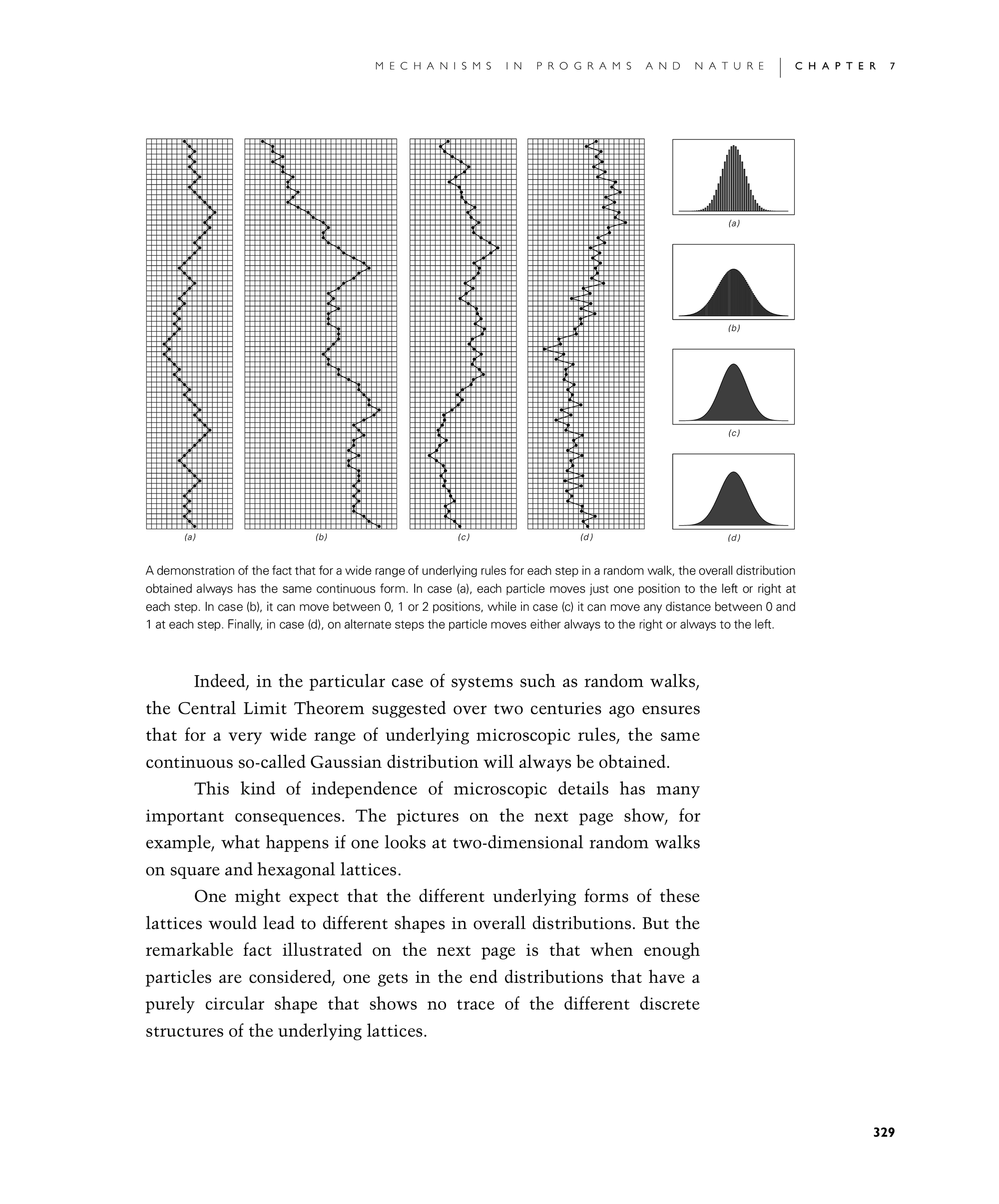
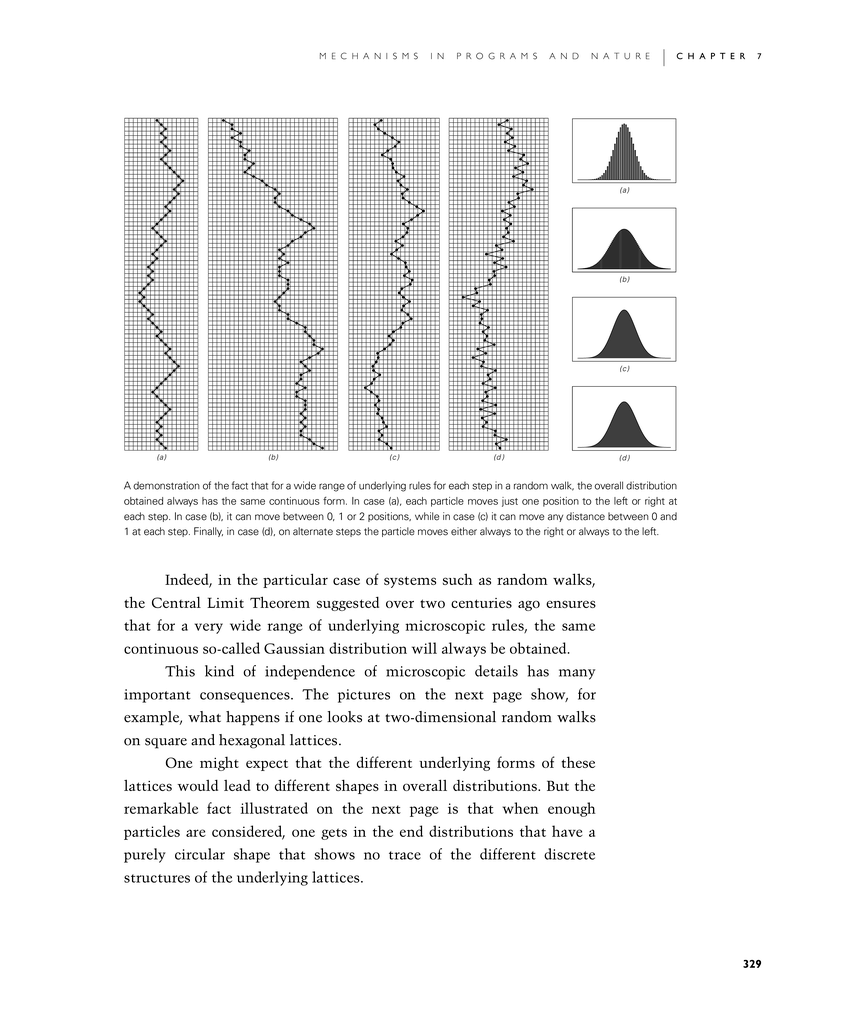
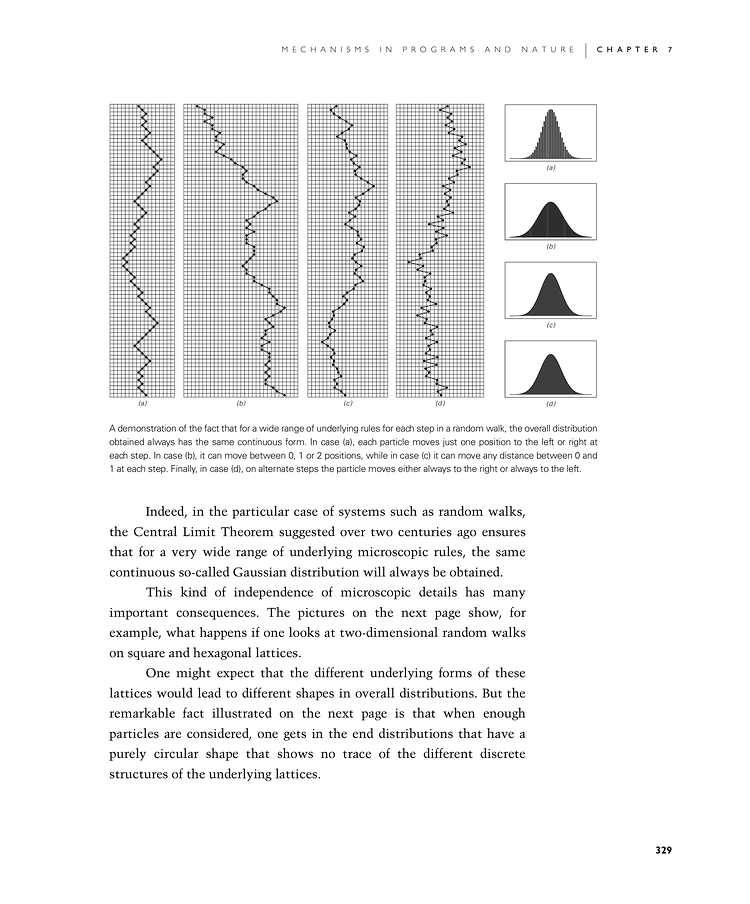
Indeed, in the particular case of systems such as random walks, the Central Limit Theorem suggested over two centuries ago ensures that for a very wide range of underlying microscopic rules, the same continuous so-called Gaussian distribution will always be obtained.
This kind of independence of microscopic details has many important consequences. The pictures on the next page show, for example, what happens if one looks at two-dimensional random walks on square and hexagonal lattices.
One might expect that the different underlying forms of these lattices would lead to different shapes in overall distributions. But the remarkable fact illustrated on the next page is that when enough particles are considered, one gets in the end distributions that have a purely circular shape that shows no trace of the different discrete structures of the underlying lattices.
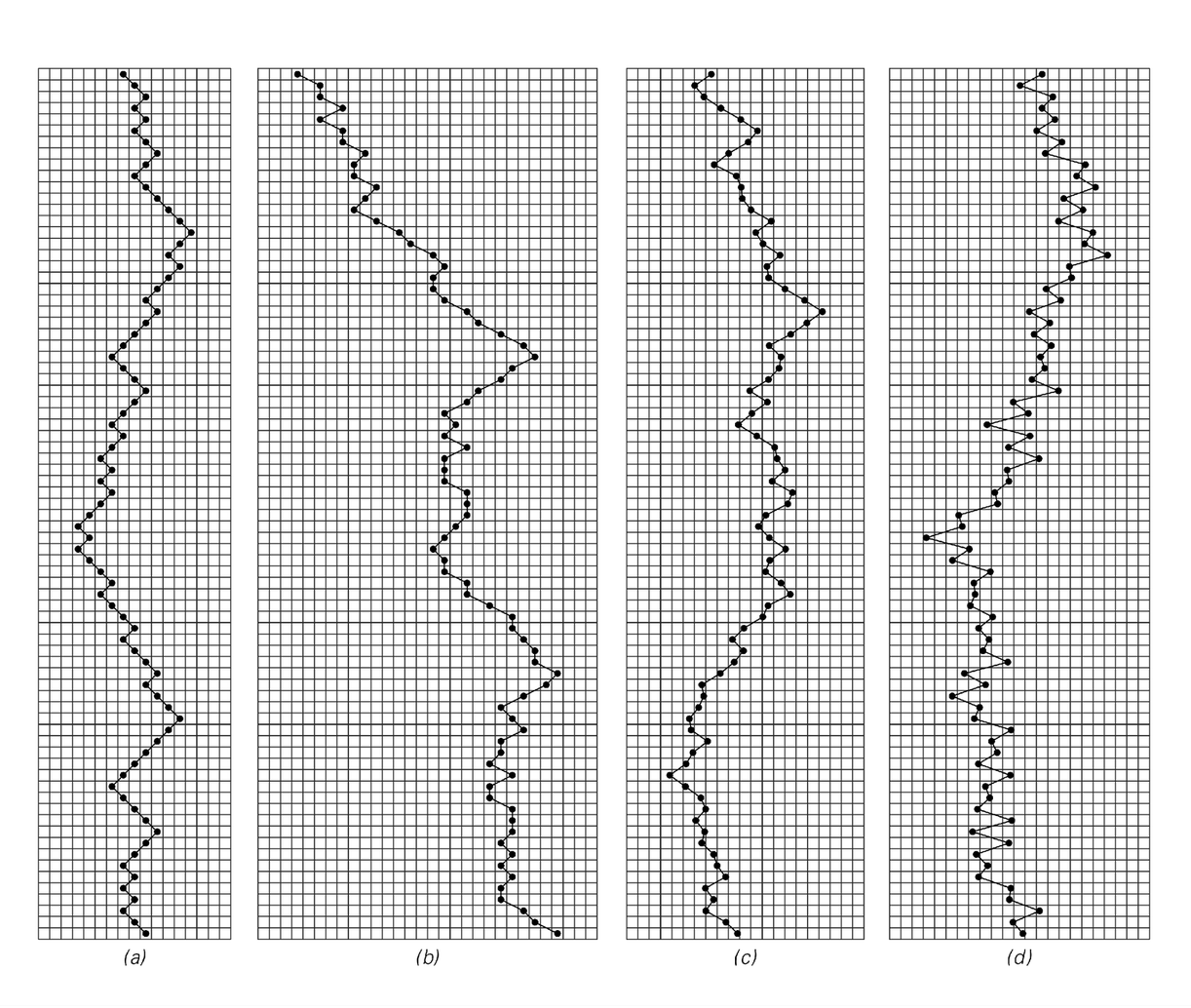 | 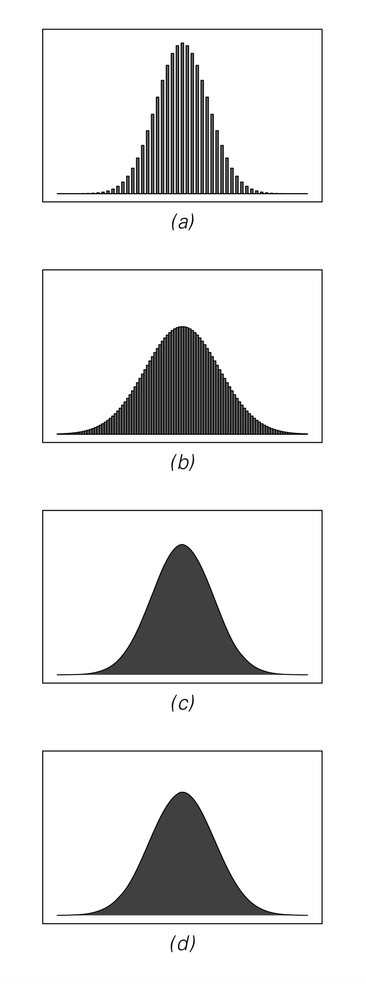 |
A demonstration of the fact that for a wide range of underlying rules for each step in a random walk, the overall distribution obtained always has the same continuous form. In case (a), each particle moves just one position to the left or right at each step. In case (b), it can move between 0, 1 or 2 positions, while in case (c) it can move any distance between 0 and 1 at each step. Finally, in case (d), on alternate steps the particle moves either always to the right or always to the left.