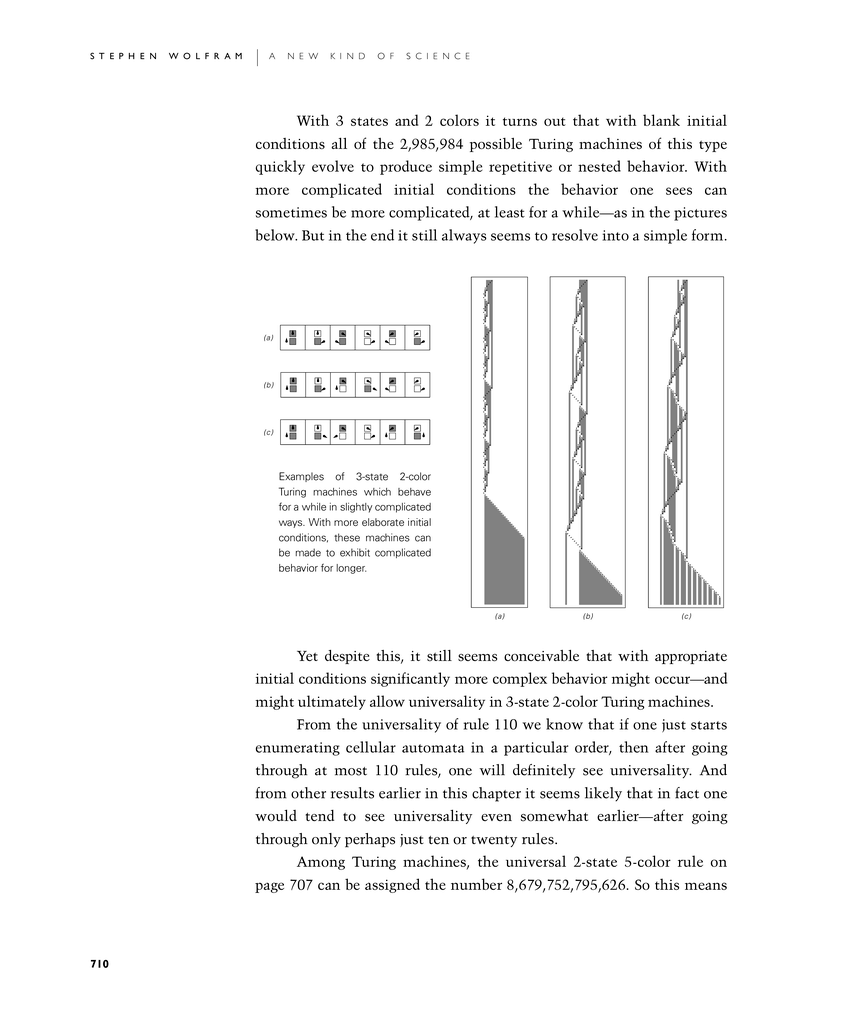
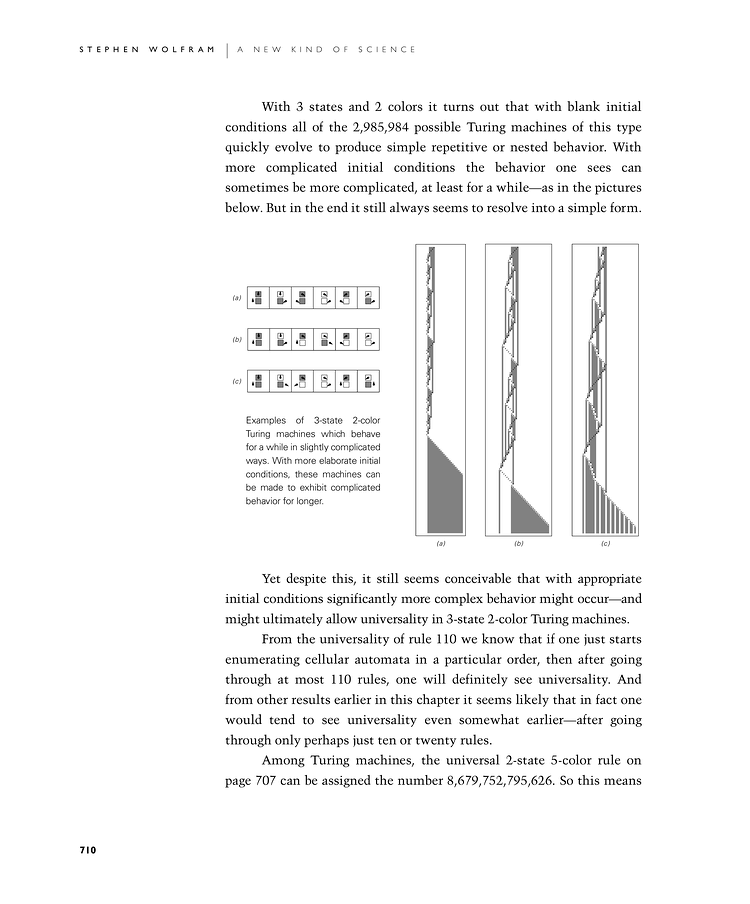
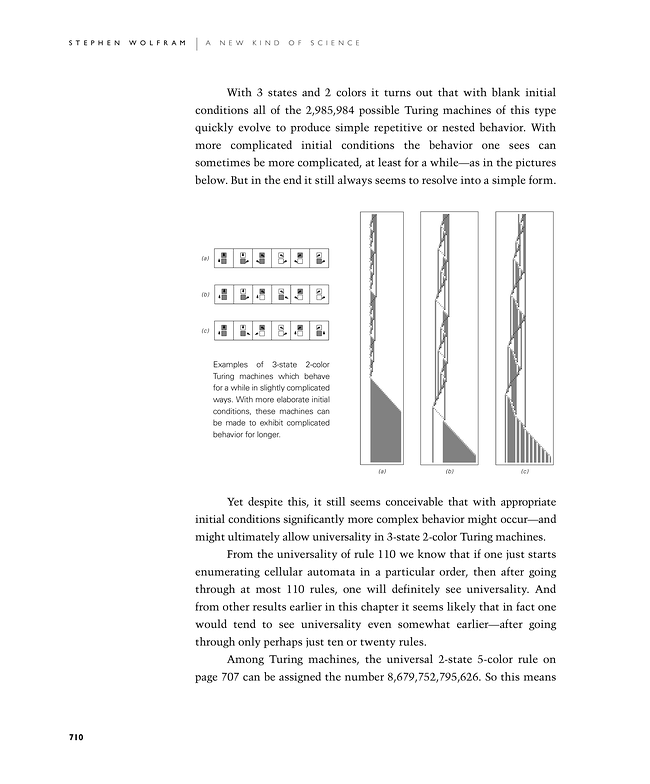
With 3 states and 2 colors it turns out that with blank initial conditions all of the 2,985,984 possible Turing machines of this type quickly evolve to produce simple repetitive or nested behavior. With more complicated initial conditions the behavior one sees can sometimes be more complicated, at least for a while—as in the pictures below. But in the end it still always seems to resolve into a simple form.

Yet despite this, it still seems conceivable that with appropriate initial conditions significantly more complex behavior might occur—and might ultimately allow universality in 3-state 2-color Turing machines.

From the universality of rule 110 we know that if one just starts enumerating cellular automata in a particular order, then after going through at most 110 rules, one will definitely see universality. And from other results earlier in this chapter it seems likely that in fact one would tend to see universality even somewhat earlier—after going through only perhaps just ten or twenty rules.

Among Turing machines, the universal 2-state 5-color rule on page 707 can be assigned the number 8,679,752,795,626. So this means

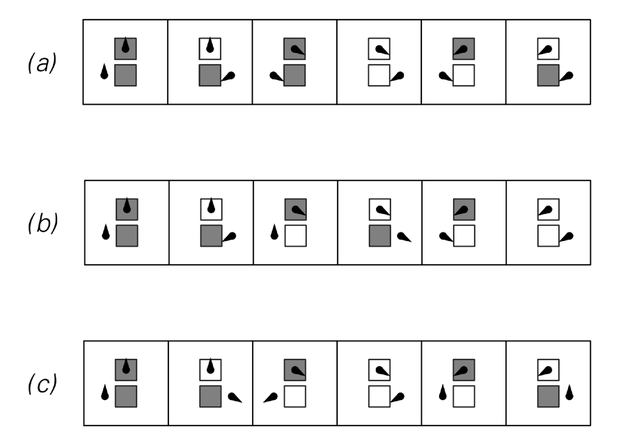Examples of 3-state 2-color Turing machines which behave for a while in slightly complicated ways. With more elaborate initial conditions, these machines can be made to exhibit complicated behavior for longer.