Notes
Chapter 8: Implications for Everyday Systems
Section 1: Issues of Modelling
https://www.wolframscience.com/nks/chap-8--implications-for-everyday-systems--notes#sect-8-1--issues-of-modelling
![Finding models [of systems]](/nks/img/thumbnails/notes-8-1--finding-models-of-systems--textonly.png "Finding models [of systems]")
Section 2: The Growth of Crystalshttps://www.wolframscience.com/nks/chap-8--implications-for-everyday-systems--notes#sect-8-2--the-growth-of-crystals
![Nucleation [of crystals]](/nks/img/thumbnails/notes-8-2--nucleation-of-crystals--textonly.png "Nucleation [of crystals]")
![Implementation [of hexagonal cellular automata]](/nks/img/thumbnails/notes-8-2--implementation-of-hexagonal-cellular-automata--textonly.png "Implementation [of hexagonal cellular automata]")
![Other models [of crystal growth]](/nks/img/thumbnails/notes-8-2--other-models-of-crystal-growth--textonly.png "Other models [of crystal growth]")
Implementation [of hexagonal cellular automata]
Other models [of crystal growth]
Section 3: The Breaking of Materialshttps://www.wolframscience.com/nks/chap-8--implications-for-everyday-systems--notes#sect-8-3--the-breaking-of-materials
![History [of fracture]](/nks/img/thumbnails/notes-8-3--history-of-fracture--textonly.png "History [of fracture]")
![Experimental data [on fracture]](/nks/img/thumbnails/notes-8-3--experimental-data-on-fracture--textonly.png "Experimental data [on fracture]")
![Alternate models [of fracture]](/nks/img/thumbnails/notes-8-3--alternate-models-of-fracture--textonly.png "Alternate models [of fracture]")
![Crushing [of solids]](/nks/img/thumbnails/notes-8-3--crushing-of-solids--textonly.png "Crushing [of solids]")
Phenomenology of microscopic fracture
Models of microscopic fracture
Experimental data [on fracture]
Alternate models [of fracture]
Section 4: Fluid Flowhttps://www.wolframscience.com/nks/chap-8--implications-for-everyday-systems--notes#sect-8-4--fluid-flow
Section 5: Fundamental Issues in Biologyhttps://www.wolframscience.com/nks/chap-8--implications-for-everyday-systems--notes#sect-8-5--fundamental-issues-in-biology
![History [of biological complexity]](/nks/img/thumbnails/notes-8-5--history-of-biological-complexity--textonly.png "History [of biological complexity]")
![Genetic programs [in biology]](/nks/img/thumbnails/notes-8-5--genetic-programs-in-biology--textonly.png "Genetic programs [in biology]")
![Tricks in [biological] evolution](/nks/img/thumbnails/notes-8-5--tricks-in-biological-evolution--textonly.png "Tricks in [biological] evolution")
![Belief in [biological] optimality](/nks/img/thumbnails/notes-8-5--belief-in-biological-optimality--textonly.png "Belief in [biological] optimality")
![Other models [of mutation]](/nks/img/thumbnails/notes-8-5--other-models-of-mutation--textonly.png "Other models [of mutation]")
![Smooth variables [in biology]](/nks/img/thumbnails/notes-8-5--smooth-variables-in-biology--textonly.png "Smooth variables [in biology]")
![[Biological] species](/nks/img/thumbnails/notes-8-5--biological-species--textonly.png "[Biological] species")
![Major new features [in biological evolution]](/nks/img/thumbnails/notes-8-5--major-new-features-in-biological-evolution--textonly.png "Major new features [in biological evolution]")
![Software [system] statistics](/nks/img/thumbnails/notes-8-5--software-system-statistics--textonly.png "Software [system] statistics")
History [of biological complexity]
Tricks in [biological] evolution
Belief in [biological] optimality
Section 6: Growth of Plants and Animalshttps://www.wolframscience.com/nks/chap-8--implications-for-everyday-systems--notes#sect-8-6--growth-of-plants-and-animals
![History [of theories of biological form]](/nks/img/thumbnails/notes-8-6--history-of-theories-of-biological-form--textonly.png "History [of theories of biological form]")
![Implementation [of branching model]](/nks/img/thumbnails/notes-8-6--implementation-of-branching-model--textonly.png "Implementation [of branching model]")
![Mathematical properties [of branching model]](/nks/img/thumbnails/notes-8-6--mathematical-properties-of-branching-model--textonly.png "Mathematical properties [of branching model]")
![Simple geometries [in branching model]](/nks/img/thumbnails/notes-8-6--simple-geometries-in-branching-model--textonly.png "Simple geometries [in branching model]")
![Projections of [phyllotaxis] patterns](/nks/img/thumbnails/notes-8-6--projections-of-phyllotaxis-patterns--textonly.png "Projections of [phyllotaxis] patterns")
![Implementation [of phyllotaxis model]](/nks/img/thumbnails/notes-8-6--implementation-of-phyllotaxis-model--textonly.png "Implementation [of phyllotaxis model]")
![Shapes of [biological] cells](/nks/img/thumbnails/notes-8-6--shapes-of-biological-cells--textonly.png "Shapes of [biological] cells")
![Symmetries [in biological systems]](/nks/img/thumbnails/notes-8-6--symmetries-in-biological-systems--textonly.png "Symmetries [in biological systems]")
![[Mollusc] shells](/nks/img/thumbnails/notes-8-6--mollusc-shells--textonly.png "[Mollusc] shells")
![[Mollusc] shell model](/nks/img/thumbnails/notes-8-6--mollusc-shell-model--textonly.png "[Mollusc] shell model")
![History [of shell models]](/nks/img/thumbnails/notes-8-6--history-of-shell-models--textonly.png "History [of shell models]")
![Discrete folding [in biological growth]](/nks/img/thumbnails/notes-8-6--discrete-folding-in-biological-growth--textonly.png "Discrete folding [in biological growth]")
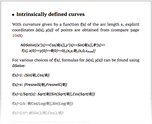
![Multidimensional generalizations [of intrinsically defined curves]](/nks/img/thumbnails/notes-8-6--multidimensional-generalizations-of-intrinsically-defined-curves--textonly.png "Multidimensional generalizations [of intrinsically defined curves]")
![Parametrizations of [biological] growth](/nks/img/thumbnails/notes-8-6--parametrizations-of-biological-growth--textonly.png "Parametrizations of [biological] growth")
![Schemes for [biological] growth](/nks/img/thumbnails/notes-8-6--schemes-for-biological-growth--textonly.png "Schemes for [biological] growth")
![[Biological] self-assembly](/nks/img/thumbnails/notes-8-6--biological-self-assembly--textonly.png "[Biological] self-assembly")
History [of theories of biological form]
Implementation [of branching model]
Mathematical properties [of branching model]
Simple geometries [in branching model]
Projections of [phyllotaxis] patterns
Implementation [of phyllotaxis model]
Symmetries [in biological systems]
Discrete folding [in biological growth]
Multidimensional generalizations [of intrinsically defined curves]
Parametrizations of [biological] growth
Section 7: Biological Pigmentation Patternshttps://www.wolframscience.com/nks/chap-8--implications-for-everyday-systems--notes#sect-8-7--biological-pigmentation-patterns
![History [of shell patterns]](/nks/img/thumbnails/notes-8-7--history-of-shell-patterns--textonly.png "History [of shell patterns]")
![Animals [with patterning] shown](/nks/img/thumbnails/notes-8-7--animals-with-patterning-shown--textonly.png "Animals [with patterning] shown")
![Implementation [of patterning model]](/nks/img/thumbnails/notes-8-7--implementation-of-patterning-model--textonly.png "Implementation [of patterning model]")
![Features of the [patterning] model](/nks/img/thumbnails/notes-8-7--features-of-the-patterning-model--textonly.png "Features of the [patterning] model")
![Scales of patterns [on animals]](/nks/img/thumbnails/notes-8-7--scales-of-patterns-on-animals--textonly.png "Scales of patterns [on animals]")
![Examples [of reaction-diffusion] in chemistry](/nks/img/thumbnails/notes-8-7--examples-of-reaction-diffusion-in-chemistry--textonly.png "Examples [of reaction-diffusion] in chemistry")
![Origins of randomness [in animal patterning]](/nks/img/thumbnails/notes-8-7--origins-of-randomness-in-animal-patterning--textonly.png "Origins of randomness [in animal patterning]")
Animals [with patterning] shown
Implementation [of patterning model]
Features of the [patterning] model
Scales of patterns [on animals]
Section 8: Financial Systemshttps://www.wolframscience.com/nks/chap-8--implications-for-everyday-systems--notes#sect-8-8--financial-systems
